Episode Summary
Hello map fans! In this episode we pick apart three very interesting map projections, one that everyone has either already heard of or seen, and two that are much newer. But not before quickly defining what a projection is in the first place.
Map Links
Mercator 1569 World Map [2:26]
Double-sided Princeton, Gott, et al Revolutionary [18:30]
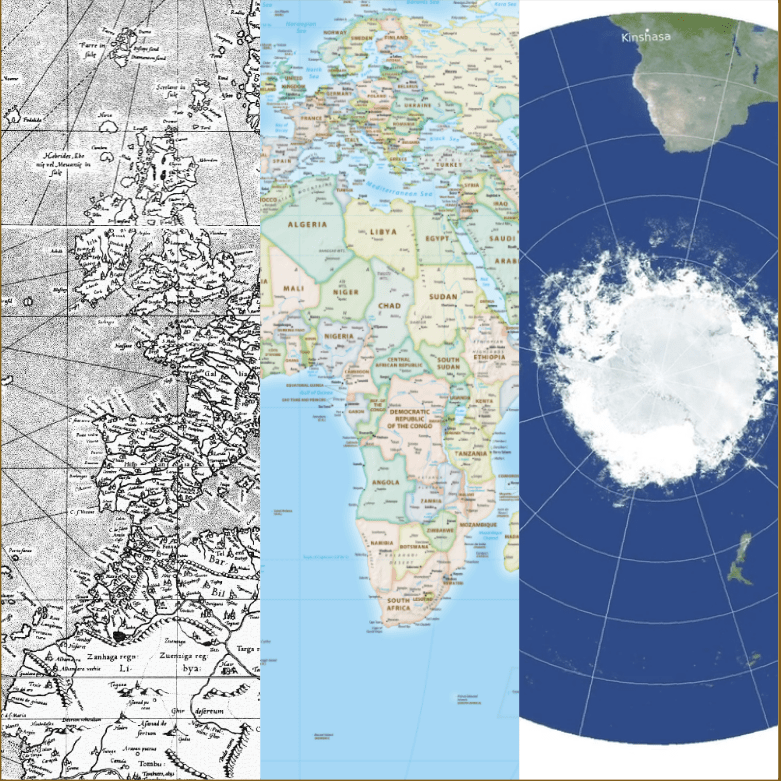
Want to visualize a 3D globe on a flat piece of paper? You have to project it first. And when you project you distort someway somehow. The three maps we discuss handily represent three different projections: Mercator, Equal-Earth, and what we're going to call "Double-Sided" in these show notes.
Mercator
Is Greenland really the same size as Africa? Mercator created his projection for a single purpose: navigation on the high seas and in particular, across the Atlantic, and this projection worked very well in getting captains and their crews from point A to point B. However, this projection is now used in online maps because of its computational ease--latitude and longitude lines form rectangles in this projection--and it isn't always good for online maps depending on their purpose.
Ever the cartographers, we also pose the question: did Mercator like or loathe writing a copyright statement on his map?
Equal-Earth
Now here's a projection that was developed just a few years ago and works well for world-scale map data. We discuss why it's good--Greenland and Africa are their actual size, for one. We bring up a few other projections like Gall-Peters [13:09], Robinson [14:53], and Winkel-Tripel [15:19] too. Wet laundry is hung up to dry metaphorically as well [16:20]. Two of these have been standards at National Geographic at varying times and we believe this will probably not be the last time that National Geographic is brought up, seeing as how they make beautiful maps.
Every projection is just trying to find the perfect accuracy and remove as much bias as possible for a particular use case, but it is hard going because any kind of flatting of the Earth will create some kind of distortion. A bad pun occurs at [15:52].
Double-Sided
This one is also called variously "The Princeton Projection" or the "Revolutionary Projection" but it does not have a standard name as of yet. If you want to read the paper that goes with this projection, as opposed to the general audience article linked to above, click here (pdf). The article refers to this projection as revolutionary and so we discuss not only what this new projection is all about but also our opinions on whether or not it is truly revolutionary. This projection is azimuthal and typically azimuthal maps, which are distorted at the equator as that is where the most stretching occurs, are set side by side but in this double-sided projection--you guessed it--they are placed on top of one another.
This was the talk of the spatial world for at least a week. Two azimuthals of the poles are not revolutionary [22:00] but technically, putting them together in this way is revolutionary [22:22]. Boundary cut off problem [23:00] is solved with this projection. A ball of clay analogy [23:20] is used to explain azimuthal projections and a party store paper lantern [26:40] analogy happens.
With maps, geography and shape really matter!

